Electric motors are the most convenient devices in use which can convert the electrical energy into mechanical energy. Electrical motors converts the electrical energy to rotational motion which can then be easily converted to any kind of mechanical motion like moving a robotic arm, moving a needle in a sewing machine etc. The Permanent Magnet Direct Current (PMDC) motors are especially useful since they are very easy to control, produce a lot of power and come in various sizes, shapes and voltage ratings.
When used with electrical or electronic circuits one must be careful about certain things like the current consuming, torque, RPM etc. This article mainly discusses those things and also the details of circuits which can be used to drive the PMDC motors.
1) MOTOR CALCULATIONS
This section is devoted for explaining the most important terms related with a PMDC motor like ‘load’, ‘torque’, ‘RPM’ etc. The capability of a PMDC motor is usually mentioned in terms of torque and RPM.
A) LOAD or MOMENT OF INERTIA
Every mass in the universe possess inertia. Inertia is the resistance of a body against its change of position, speed etc. The rotating bodies also possess a kind of inertia called moment of inertia. The moment of inertia can be considered as the rotational load or the mass equivalent in linear motion. In case of a robotic arm the rotational load is the sum of frictional force, weight of the arm etc.
Load or moment of inertia is equal to the amount of rotational force that should be applied to just start rotating a body which was at rest
B) TORQUE & CURRENT
Torque of a motor is the rotational force which it should generate so as to accelerate a Load attached to its shaft to a required rotating speed.
In the case of a motor the rotating body could be anything like a robotic arm, wheels of a toy car etc. If the motor is running with the rotating body at the required speed then it can be said that the motor is generating enough torque as required.The relation between the torque and the load is shown in the following equation;
TORQUE = LOAD * ANGULAR ACCELERATION
If the torque is represented by ‘T’, the load is represented by ‘L’ and the angular acceleration as ‘A’, then the above equation cab be re-written as
T = L * A
When a motor accelerates a load ‘L’ from 0 RPM to a constant RPM within ‘t’seconds, then the torque generated can be found as
![]()
Torque is actually a kind of force whose value increases as the distance from the center of rotation increases. Consider the below figure in which a rolling wheel is shown which is driven by a motor.
[sam id=”5″ codes=”true”]
The radius of a wheel is marked in the figure and the force required to rotate it is also marked in red. This wheel has its own weight and there might be weight of a vehicle adding to that if the wheel is attached to one. Now the torque required to rotate the wheel can be calculated using the following relation,
T = FD
Where;
T is the torque required,
F is the amount of force that should be applied and
D is the distance from the center of the shaft to the point where the torque is measured, in the case of a wheel like the one shown in the above figure the distance is the radius of the wheel itself.
Hence simply by increasing the diameter of the wheel the torque can be increased.The unit of the torque is Newton Meter (Nm)
Thetorque ‘T’ which a dc motor generates to rotate a body and the current ‘I’ consuming at the same time has a linear relationship. The value of the torque and the current consuming is always proportional to each other and the proportionality constant is called the torque constant KT.
T = KT * I
The value of the KTmight be available in the datasheet of a DC motor or else it can be calculated from the value of other parameter given in the datasheet like NOMINAL TORQUE and NOMINAL CURRENT, which are actually the normal values of torque and current at which the motor is supposed to operate.
The torque constant can be calculated as;
KT = NOMINAL TORQUE / NOMINAL CURRENT
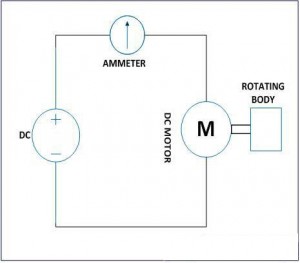
Once the value of the KT is found then the torque generated by the motor while it is running at any value of current can be calculated by using the relation T = KT* I. The following arrangement shows how it is possible to calculate the torque generated by a motor using a simple multi-meter;
If a wheel is used as the rotating body then the simplest way to vary the torque requirement is to increase the mass of the rotating body by increase the number of wheels attached to the shaft. More the number of wheels more is the requirement of the torque and the motor should generate that much torque
RATED VOLTAGE in the datasheet of the motor. The RATED VOLTAGE is the voltage at which the motor is designed to operate and all the values of the datasheet have been measured at the same voltage.
In the circuit an ordinary multi-meter can be used in power measuring mode as an ammeter which will display the value of current consumed by the motor at different loads.
Suppose if the value of the KT has been calculated from the datasheet using the relation
KT= NOMINAL TORQUE / NOMINAL CURRENTas 0.003 and the current consuming by the motor while running at the RATED VOLTAGEwith an unknown load is 10mA, the torque generated by the motor can be calculated as follows
T = 0.003 * 0.01 = 0.03 mNm
C) RPM &VOLTAGE
The speed of a motor is usually mentioned in terms of RPM (Rotationsper Minute). There is a relation between the RPM, voltage applied and the current consuming for a particular motor.
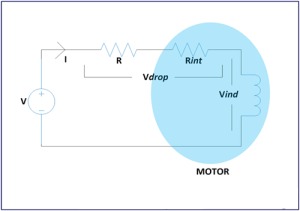
Consider the following figure in which the internal equivalent circuit of the motor running at a DC supply of ‘V’ is shown;
The internal armature of a motor can be viewed as having an ideal coil with an ideal series resistance. They are mentioned ideal since the coil is purely inductive and should not introduce any resistance into the circuit. The internal resistance is purely resistive and should not introduce any inductance into the circuit. In the circuit shown above the internal resistance is marked as ‘Rint’ and the voltage induced across the internal inductance is ‘Vind’. This is the equivalent of the motor armature or winding coil.
Vind = V – Vdrop
Now the significance of this circuit is that the speed of the motor depends purely on the voltage ‘Vind’ and the torque generated at that voltage depends purely on the value of current ‘I’ flowing through circuit.
It is already discussed previously that the torque is always proportional to the current flowing through the circuit by a proportionality constant KT. Similarly the speed and the voltage induced ‘Vind’ is also proportional to each other by the same constant.
Vind = KE * S
Where;
Vind is the induced voltage in the coil
S is the speed in the unit of radians/seconds
KE is the voltage constant which has the same value as KT
From the above equation the speed in RPM can be calculated as

Since the Vind can’t be measure directly since in real the ‘Rint’ and the coil together forms the winding coil of the armature. Since the same current ‘I’ flows through the resistance and the coil the following equation is more useful in finding the speed in RPM with respect to the applied voltage ‘V’.

The ‘V’ is the constant DC voltage supplied, the Rint is a constant which might be given in the datasheet as COIL RESISTANCE or can be measure using a multi-meter, ‘KE‘which is same as ‘KT‘ can be calculated as discussed in the previous section and the ‘R’ is a known value of resistance.The following circuit can be used to do the calculate the speed of a motor at different torquerequirement and at different values of ‘R’;
It can be found that as the torque requirement increases the current ‘I’ increases. Hence for a particular value of current ‘I’, using the previous equation for calculating speed;
RPM = ( V – I ( R + Rint ) ) * 60 / ( 2? * KE )
The RPM decreases as the value of ‘R’ increases and as the value of ‘R’ decreases the RPM increases. Hence using the above circuit the RPM for a motor running with a load can be adjusted.
D) POWER
The power is the capability of a motor to keep on rotating a Load at a particular speed
The power is defined as the product of the torque and the speed. If the speed is calculated in RPM the the power is given by the equation
![]()
Where;
P is the power calculated
T is the torque generated
RPM is the speed of the motor
Summary:
· Load or moment of inertia is equal to the just enough amount of rotational force that should be applied to start rotating a body which was at rest
· Torque of a motor is the rotational force which it should generate so as to accelerate a Load attached to its shaft to a required rotating speed
When a motor accelerates a load ‘L’ from 0 RPM to a constant RPM within ‘t’ seconds, then the torque generated can be found as
![]()
· The torque generated by a motor depends only on the current flowing through the motor. As the demand for the torque increases the current flow increases as per the equation
T = KT * I
· The speed of a motor depends only on the voltage induced across the coil
![]()
· As the current increases due to the torque the RPM decreases as per the equation
![]()
· The power is the capability of a motor to keep on rotating a Load at a particular speed
![]()






Post Comment
You must be logged in to post a comment.